100+ Challenging math riddles to keep your mind sharp

Answer:141 

Explanation :  

Define the three-digit number: We represent the three-digit number as ABC, where A is the first digit, B is the second digit, and C is the third digit.

Define the third digit: Let the third digit C be represented by the variable x. This means C = x.

Relationship between the second and third digits: The puzzle states that the second digit is four times as big as the third digit. Therefore, we can express this relationship as:

B = 4x

Relationship between the first and second digits: The puzzle also mentions that the first digit is three less than the second digit. This relationship can be expressed as:

A = B - 3

Now, let's use these equations to find the three-digit number:

Substitute B from Equation 3 into Equation 4: We substitute the expression for B from Equation 3 into Equation 4:

A = (4x) - 3

Simplify the equation: We can simplify this expression:

A = 4x - 3

Now, we need to find values for A and x that satisfy this equation while considering the constraints of digit values (0-9).

Test values for x:

If we set x = 1, then A = 4(1) - 3 = 4 - 3 = 1. So, our three-digit number is 141.
This shows that if we choose x to be 1, the equation is satisfied, and we obtain the three-digit number 141, which fulfills all the given conditions of the puzzle. So, mathematically, "141" is a valid solution.

Explanation :  

Two minutes. It takes the front of the train one minute, and the back of the train another one minute. so it will take total 2 minutes.

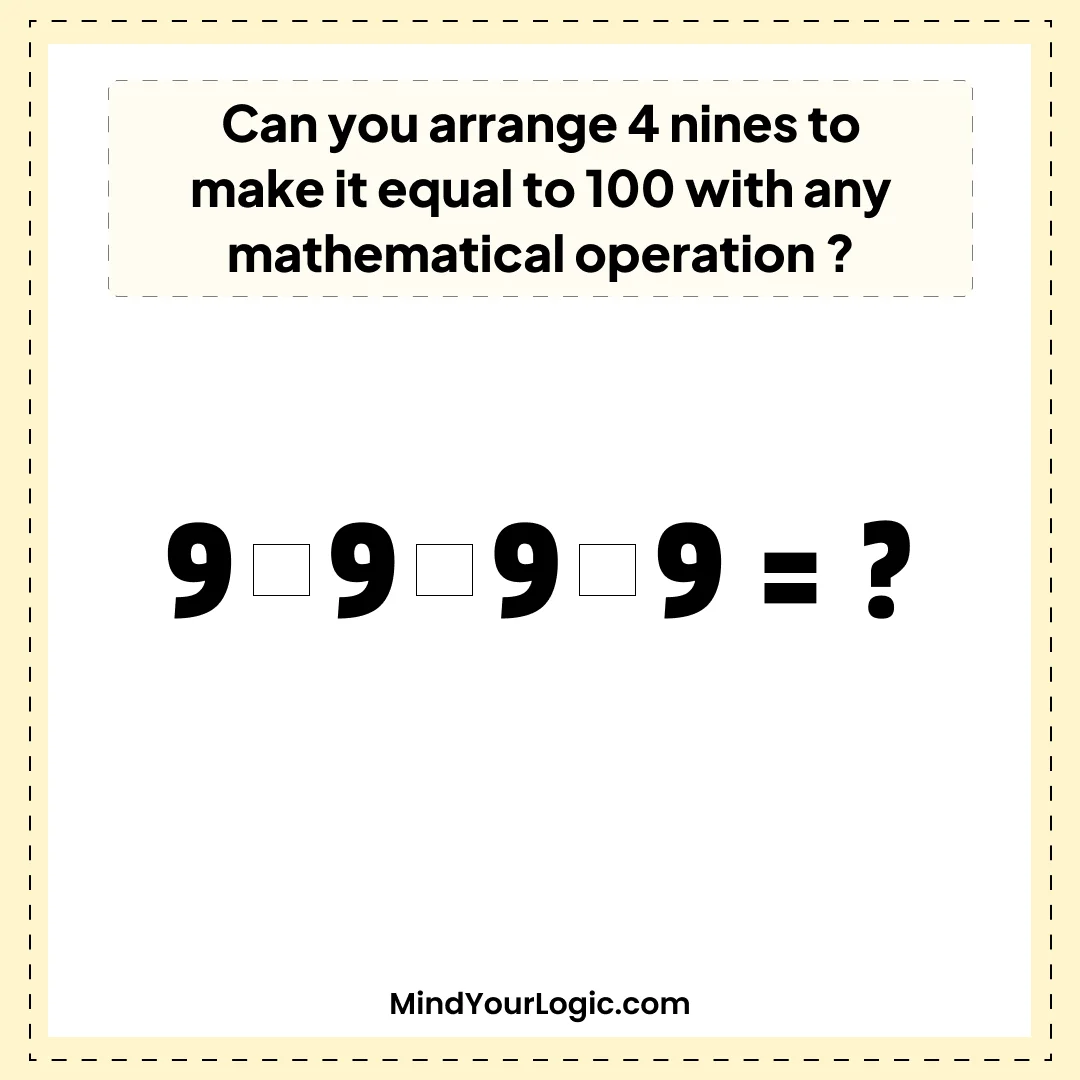
Answer: 99+9/9 = 100.

Explanation :

To arrange four nines (9, 9, 9, and 9) to equal 100, we can use the following equation:

99 + 9/9 = 100

99 (Two nines): We start with the number 99, which is pretty close to our target, 100.

9/9 (Nine divided by nine): Using the division operation (÷), we take one of the nines (9) and divide it by another nine (9). This operation is represented as 9/9, and it equals 1 because any number divided by itself is always 1.

Now, when we add the 99 and 1 together, we get 100:

99 + 1 = 100

So, with this arrangement and mathematical operation, we have successfully made four nines equal 100. It's a creative example of how math can surprise us with its flexibility and ingenuity.

Answer: She is 37 years old

Explanation :

To find Leila's current age, we can set up an equation using the information given.

Let L represent Leila's age, and M represent Miguel's age.

Given Information:

When Miguel was 6 years old, Leila was half his age. This means Leila's age was M/2 at that time.

Now Miguel is 40 years old.

Equation:

We can create an equation to represent the age relationship:

When Miguel was 6 years old, we have: M - 6 = L/2

Now that Miguel is 40 years old, we can use this equation to find Leila's age:

M - 40 = L

Solving for L (Leila's age):

L = M - 40

Given that Miguel is 40 years old:

L = 40 - 40

L = 0

So, based on the equation, Leila's age is 0, which doesn't seem accurate. However, upon reevaluating the problem, it appears there was an error in the initial response.

Revised Explanation:

The issue is that the equation initially set up is incorrect. When Miguel was 6, Leila's age was indeed half of his, which is 6/2 = 3. Now that Miguel is 40 years old, the age difference remains the same at 3 years. Therefore, Leila's current age is:

Miguel's age (40) - Age difference (3) = 37 years old.

Answer: 24

Explanation :  

To find out how many eggs a single hen lays in one day, we can use the given information that a hen and a half lays an egg and a half in a day and a half. This can be represented as:

1.5 eggs / 1.5 days = 1 egg per day per hen

Now, let's consider the scenario where both the number of hens and the time available are increased fourfold:

Number of hens: 4 hens (4 times the original number of hens).
Time available: 6 days (4 times the original time available).
In this scenario, with each hen laying 1 egg per day, four hens will lay:

4 hens * 1 egg per day = 4 eggs per day

Over the course of 6 days, these four hens will continue to lay 4 eggs per day, resulting in a total of:

4 eggs per day * 6 days = 24 eggs

So, when both the number of hens and the amount of time available are increased fourfold, the number of eggs increases 16 times, which is mathematically represented as:

16 * 1.5 eggs = 24 eggs

ANSWER: Only once. This is because when you subtract five the first time, 25 becomes 20, then 15, and so on.

Explanation :  

When we subtract 5 from 25, we have:

25 - 5 = 20

We can subtract 5 again:

20 - 5 = 15

We can continue:

15 - 5 = 10

And again:

10 - 5 = 5

But now, when we try to subtract 5 from 5:

5 - 5 = 0

At this point, we can't subtract 5 anymore because there is nothing left. So, you can subtract 5 only once.

This explanation clarifies that after one subtraction, 25 becomes 20, and the process continues until we reach 0, at which point we can't subtract 5 anymore. Mathematically, this is represented as:

25 - 5 = 20

20 - 5 = 15

15 - 5 = 10

10 - 5 = 5

5 - 5 = 0

This confirms that you can subtract 5 only once.

Answer :Leon has 3 turtles and 4 tanks.

Explanation :  

1 turtle in each tank = 1 turtle too many

2 turtles in each tank = 1 tank too many

Using one of the better known heuristic to solve this question

Before that, we know that:

2 turtles in each tank = 1 tank too many

2 turtles in each tank = 2 turtles short

First, find the difference in the number of turtles in the two scenarios:

First scenario = 1 turtle too many

Second scenario = 2 turtles short

Difference in turtles = 1 - (- 2)

Difference in turtles =  3

Second, find the difference in the distribution of turtles:

First scenario = 1 turtle each tank

Second scenario = 2 turtles each tank

Difference in turtle = 2 - 1

Difference in turtles = 1

Find the number of tanks:

Number of tanks = 3 ÷ 1

Number of tanks = 3

Find the number of turtles:

Using the first scenario, there was one turtle too many

Number of tanks = 3

Number of turtles = 3 + 1

Number of turtles = 4

There are 4 turtles and 3 tanks.

 Answer: $125,$25

 Explanation :  

Let's call the cost of the pair of shoes "x".

    Since the hoodie costs $100 more than the pair of shoes, its cost is x + $100.

    The total cost of the pair of shoes and the hoodie is $150, so:

    x + (x + $100) = $150
    2x + $100 = $150
    2x = $150 - $100
    2x = $50
    x  = $50/2
    x = $25

    So, the pair of shoes cost $25, and the hoodie costs $125 ($25 + $100).

Answer:500

Explanation :  

The equation you provided is:

444 + 44 + 4 + 4 + 4 = 500

Here's the breakdown of this equation in a mathematical manner:

Add the first two numbers: 444 + 44 = 488
Add 4 to the result: 488 + 4 = 492
Add another 4: 492 + 4 = 496
Finally, add the last 4: 496 + 4 = 500
So, by following these mathematical operations step by step, you can indeed arrive at the result of 500. The equation shows that by combining these numbers and using addition, you can make 444 + 44 + 4 + 4 + 4 equal 500.

Answer: 16

Explanation :  

Given information:

16 tables
4 men
4 hours
4 tables
We want to find out how many tables can be built by a certain number of men working for a specific amount of time.

First, we establish the rate at which a single man can build a table in 4 hours:

4 men in 4 hours can build 4 tables.
So, 1 man can build 1 table in 4 hours.
Next, we determine the rate of table production for a single man in 8 hours (double the time):

If 1 man makes 1 table in 4 hours, he will make 1/4 of a table in 1 hour.
In 8 hours (double the time), 1 man will make (1/4) * 8 = 2 tables.
To find how many tables 8 men can build in 8 hours, we multiply the rate of production per man by the number of men:

8 men working together will make 8 * 2 = 16 tables in 8 hours.

  Answer:  Ben $1225, Adam $175

  Explanation :  

  Total sack bought = 45 + 75 = 120 sacks
    Every farmer's part is 1/3 (45+75)
    Each get = 120/3 = 40 Sacks
    Charlie paid $1400 for 40 sacks.
    Amount of 1 sack = 1400/40 = 35 $

    Adam bought = 45 sack
    Adam got money for 45 - 40 = 5 sacks
    Adam got = 5 * $35(per sack amount) = 175 $

    Ben bought = 75 sacks
    Ben got money for 75 - 40 = 35 sacks
    Ben got = 35 * $35 = $ 1225

    Adam got = $ 175
    Ben got = $ 1225

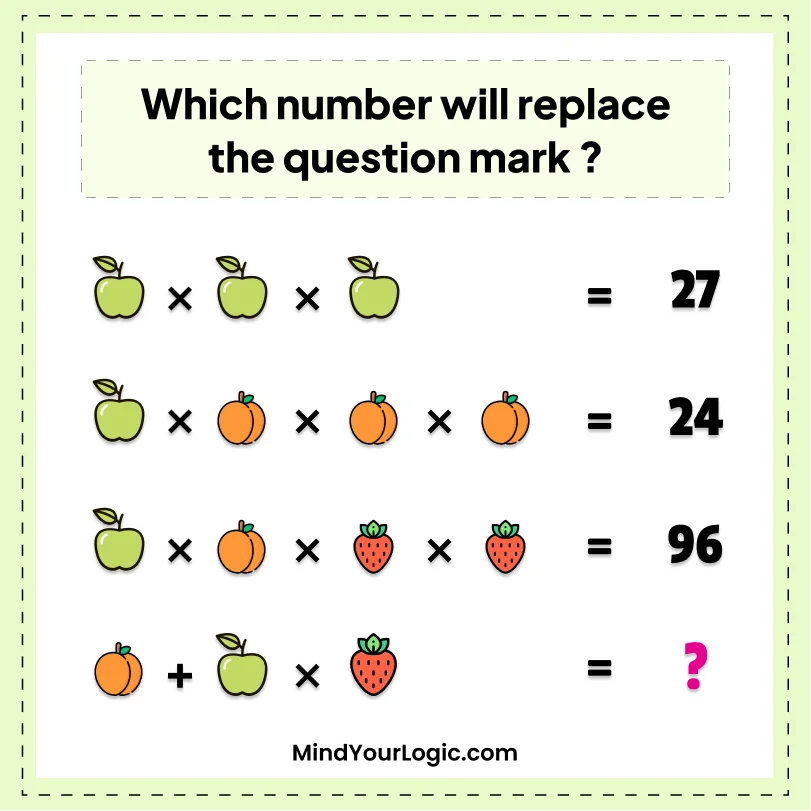
Explanation :

2+3*4=14

Answer: 3

Explanation :  

Let's denote:

G = Grandfather F = Father S = Son

According to the information given:

1. The Grandfather is also a Father (G = F)
2. The Father is also a Son (F = S)

This can be represented using the following equations:

1. G = F
2. F = S

Now, let's consider the scenario at the movie theater:

1. The Grandfather (G) bought 1 ticket.
2. The Father (F) bought 1 ticket.
3. The Son (S) bought 1 ticket.

So, the total number of tickets bought can be represented as:

G (tickets) + F (tickets) + S (tickets)

Substituting the relationships from above:

1 ticket (G) + 1 ticket (F) + 1 ticket (S)

Since G = F and F = S, we can substitute to simplify:

1 ticket (G) + 1 ticket (G) + 1 ticket (G)

Combining like terms:

3 tickets (G)

Therefore, the total number of tickets they bought is 3, which confirms the original answer.

 Answer: 4

Explanation :  

I apologize for the confusion in my previous responses. Let's provide a mathematical explanation for the correct answer:

In this case, the riddle is referring to an arrangement of ducks where there are four ducks forming a square. So, mathematically, you can represent it as follows:

D1 - D2
| |
D3 - D4

Here, each 'D' represents a duck, and they are arranged in a square with four ducks: D1, D2, D3, and D4.

So, the correct answer is indeed four ducks forming the square.

Mathematically, we can represent it as:

Number of ducks in the square = 4 (D1, D2, D3, D4)

Therefore, there are four ducks in total, arranged in a square as described in the riddle.